A significant advancement in quantum computing has emerged with the development of a novel error-correction technique that allows quantum computers to dynamically switch between different error correction codes during computations. This innovation addresses one of the fundamental challenges in quantum computing: maintaining accuracy while performing complex calculations.
The core challenge in quantum error correction stems from a fundamental difference between classical and quantum computing. While classical computers can simply create multiple copies of data to check for errors, quantum systems cannot duplicate quantum states due to the laws of quantum mechanics. This limitation has necessitated the development of specialized approaches to error correction in quantum systems.
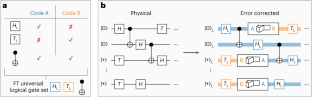
The quantum error correction method works by distributing information across multiple entangled quantum bits (qubits), creating a redundancy that enables error detection and correction. This approach, while effective, has faced limitations due to a fundamental theorem in quantum computing which states that no single correction code can efficiently perform all necessary gate operations while maintaining error protection for fully programmable computations.
The breakthrough came from a collaboration between researchers at the University of Innsbruck and RWTH Aachen, along with the Peter Grünberg Institute at Forschungszentrum Jülich. Building on their 2022 success in implementing fault-tolerant quantum bits, the team, led by Thomas Monz and Markus Müller, developed a method allowing quantum computers to alternate between two different correction codes in an error-tolerant manner.
This dual-code approach provides a practical solution to the limitations of single-code systems. When the quantum computer encounters a logic gate that is difficult to implement in the first code, it can switch to the second code, making the overall computation more efficient and reliable. This flexibility in code switching represents a significant step forward in quantum computing capability.
The implementation of this theoretical framework was achieved through close collaboration between the research teams. Friederike Butt, a doctoral student in Müller’s group, developed the quantum circuits that formed the foundation of the experiment. These circuits were then implemented in practice through collaboration with Monz’s research group in Innsbruck, where PhD student Ivan Pogorelov played a key role in the experimental work.
The successful implementation marks the first time researchers have achieved a universal set of quantum gates on an ion trap quantum computer using combined quantum error correction codes. This achievement builds on the long-standing collaboration between Monz and Müller, whose professional relationship dates back to their time as doctoral students at the University of Innsbruck.
This advancement represents a crucial step forward in making quantum computers more practical and reliable. The ability to switch between error correction codes on-the-fly addresses one of the major obstacles in quantum computing: maintaining computational accuracy while performing complex operations. This breakthrough could accelerate the development of more sophisticated quantum computing applications and bring us closer to realizing the full potential of quantum computers.
The research findings, published in Nature Physics, demonstrate how theoretical insights in quantum computing can be successfully translated into practical implementations. This work exemplifies the importance of collaboration between theoretical and experimental quantum physics teams in advancing the field of quantum computing.
This development is particularly significant as it shows how quantum computers can overcome inherent limitations through innovative approaches to error correction, potentially paving the way for more complex and reliable quantum computations in the future.
Reference: “Experimental fault-tolerant code switching” by Ivan Pogorelov, Friederike Butt, Lukas Postler, Christian D. Marciniak, Philipp Schindler, Markus Müller and Thomas Monz. 24 January 2025, Nature Physics. DOI: 10.1038/s41567-024-02727-2