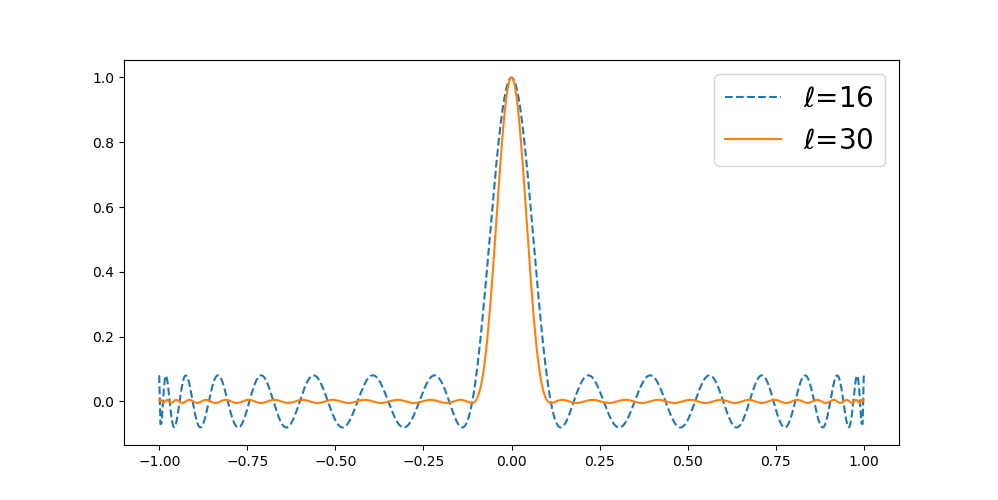
Researchers at Berkeley present a quantum eigenstate filtering algorithm that allows to efficiently prepare a target eigenstate of a given Hamiltonian to high precision under reasonable assumptions.
They have applied this algorithm to the quantum linear system problem, and present two algorithms based on quantum adiabatic computing and quantum Zeno effect respectively.
Both algorithms prepare the final solution as a pure state, and achieves the near optimal query complexity. Neither algorithm uses phase estimation or amplitude amplification.
