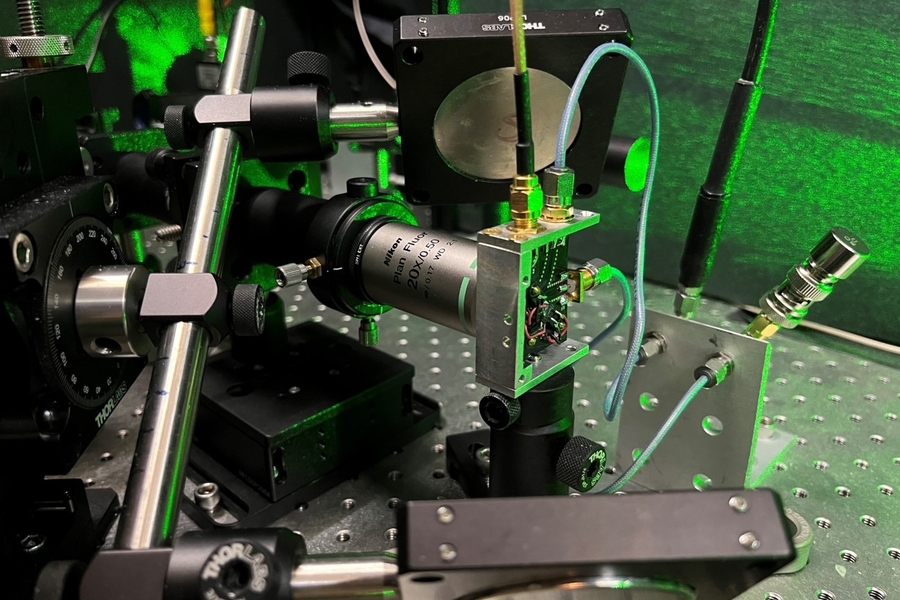
Researchers at MIT developed a method to enable quantum sensors to detect any arbitrary frequency, with no loss of their ability to measure nanometer-scale features.
Quantum sensors detect the most minute variations in magnetic or electrical fields, but until now they have only been capable of detecting a few specific frequencies, limiting their usefulness.
Quantum sensors can take many forms; they’re essentially systems in which some particles are in such a delicately balanced state that they are affected by even tiny variations in the fields they are exposed to. These can take the form of neutral atoms, trapped ions, and solid-state spins, and research using such sensors has grown rapidly. For example, physicists use them to investigate exotic states of matter, including so-called time crystals and topological phases, while other researchers use them to characterize practical devices such as experimental quantum memory or computation devices. But many other phenomena of interest span a much broader frequency range than today’s quantum sensors can detect.
The new system the team devised, which they call a quantum mixer, injects a second frequency into the detector using a beam of microwaves. This converts the frequency of the field being studied into a different frequency — the difference between the original frequency and that of the added signal — which is tuned to the specific frequency that the detector is most sensitive to. This simple process enables the detector to home in on any desired frequency at all, with no loss in the nanoscale spatial resolution of the sensor.
In their experiments, the team used a specific device based on an array of nitrogen-vacancy centers in diamond, a widely used quantum sensing system, and successfully demonstrated detection of a signal with a frequency of 150 Megahertz, using a qubit detector with frequency of 2.2 Gigahertz — a detection that would be impossible without the quantum multiplexer. They then did detailed analyses of the process by deriving a theoretical framework, based on Floquet theory, and testing the numerical predictions of that theory in a series of experiments.
The paper has been published in the journal Physical Review X.