npj Quantum Information, Published online: 21 November 2024; doi:10.1038/s41534-024-00901-1
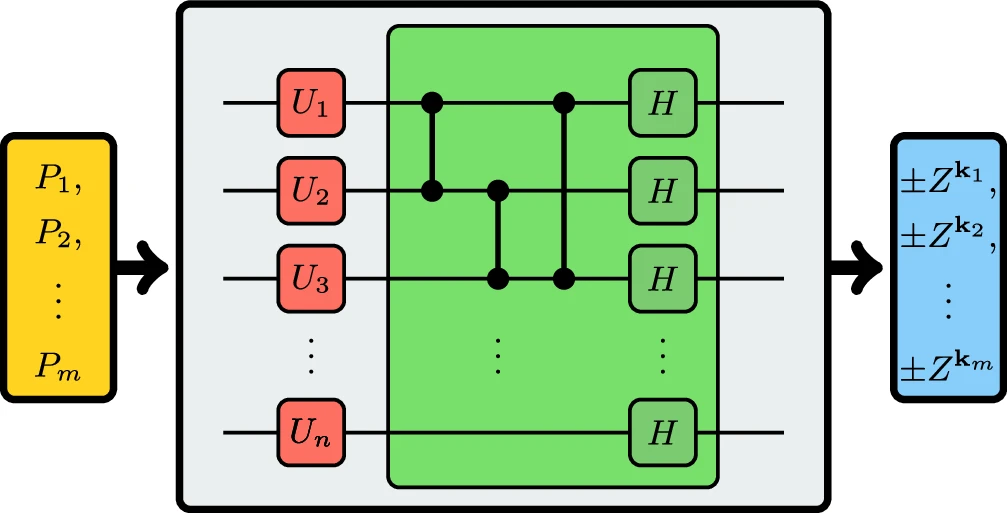
A central building block of many quantum algorithms is the diagonalization of Pauli operators. Although it is always possible to construct a quantum circuit that simultaneously diagonalizes a given set of commuting Pauli operators, only resource-efficient circuits can be executed reliably on near-term quantum computers.
Generic diagonalization circuits, in contrast, often lead to an unaffordable SWAP gate overhead on quantum devices with limited hardware connectivity. A common alternative is to exclude two-qubit gates altogether. However, this comes at the severe cost of restricting the class of diagonalizable sets of Pauli operators to tensor product bases (TPBs).
A team of researchers have introduced a theoretical framework for constructing hardware-tailored (HT) diagonalization circuits. Their framework establishes a systematic and highly flexible procedure for tailoring diagonalization circuits with ultra-low gate counts. They have highlighted promising use cases of our framework and – as a proof-of-principle application – devised an efficient algorithm for grouping the Pauli operators of a given Hamiltonian into jointly-HT-diagonalizable sets.
For several classes of Hamiltonians, they observed that this approach requires fewer measurements than conventional TPB approaches.
Finally, they experimentally demonstrated that HT circuits can improve the efficiency of estimating expectation values with cloud-based quantum computers.